A Computational Design Method for Tucking Axisymmetric Origami Consisting of Triangular Facets
Yan Zhao1*, Yuki Endo2, Yoshihiro Kanamori3, and Jun Mitani3
1 Jiangsu University
2 Toyohashi University of Technology
3 University of Tsukuba
Abstract:
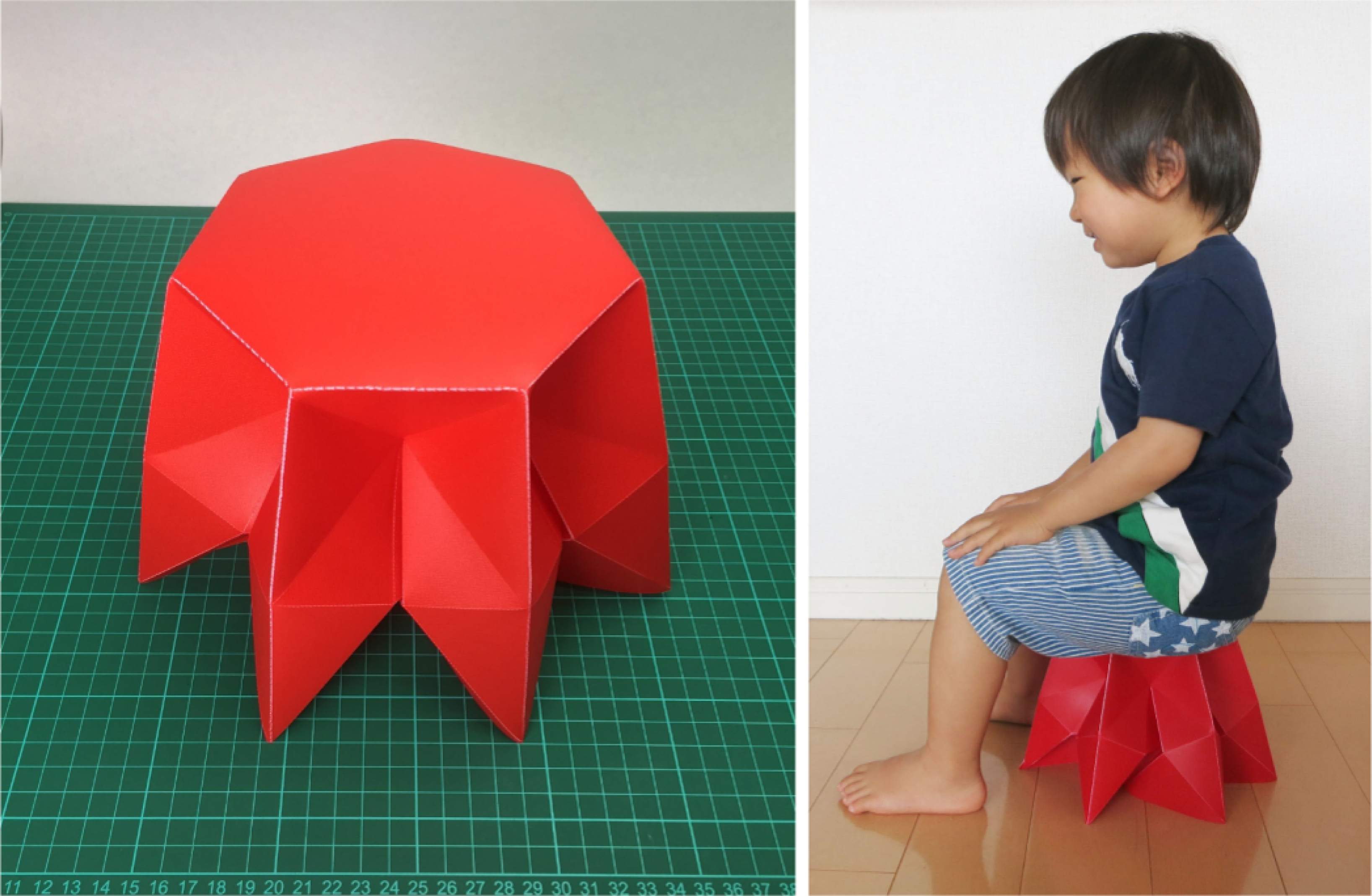
Three-dimensional (3D) origami, which can generate a structure through folding a crease pattern on a flat sheet of paper, has received considerable attention in art, mathematics, and engineering. With consideration of symmetry, the user can efficiently generate a rational crease pattern and make the fabricated shape stable. In this paper, we focus on a category of axisymmetric origami consisting of triangular facets and edit the origami in 3D space for expanding its variations. However, it is difficult to retain the developability, which requires the sum of the angle around each interior vertex should equal 360 degrees, for designing an origami. Intersections occur between crease lines when such a value is larger than 360 degrees. On the other hand, blank spaces (unfolded areas) emerge in the crease pattern when the value is less than 360 degrees. The former case is difficult to generate a realizable shape due to the crease lines are intersected with each other. For the latter case, however, blank spaces can be filled with crease lines and become a part of the origami through trucking. Here, we propose a computational method to add flaps or tucks on the 3D shape, which contains non-developable interior vertices, for achieving the resulting origami. Finally, on the application side, we describe a load-bearing experiment on a stool shape-like origami to demonstrate the potential usage.
Prototype system
Results
Publications:
- Yan Zhao*, Yuki Endo, Yoshihiro Kanamori, Jun Mitani: "A Computational Design Method for Tucking Axisymmetric Origami Consisting of Triangular Facets", Symmetry 2018, 10(10), 469. (SCIE IF: 1.213).
BibTeX Citation
AUTHOR = {Zhao, Yan and Endo, Yuki and Kanamori, Yoshihiro and Mitani, Jun},
TITLE = {A Computational Design Method for Tucking Axisymmetric Origami Consisting of Triangular Facets},
JOURNAL = {Symmetry},
VOLUME = {10},
YEAR = {2018},
NUMBER = {10},
ARTICLE-NUMBER = {469},
DOI = {10.3390/sym10100469}
}